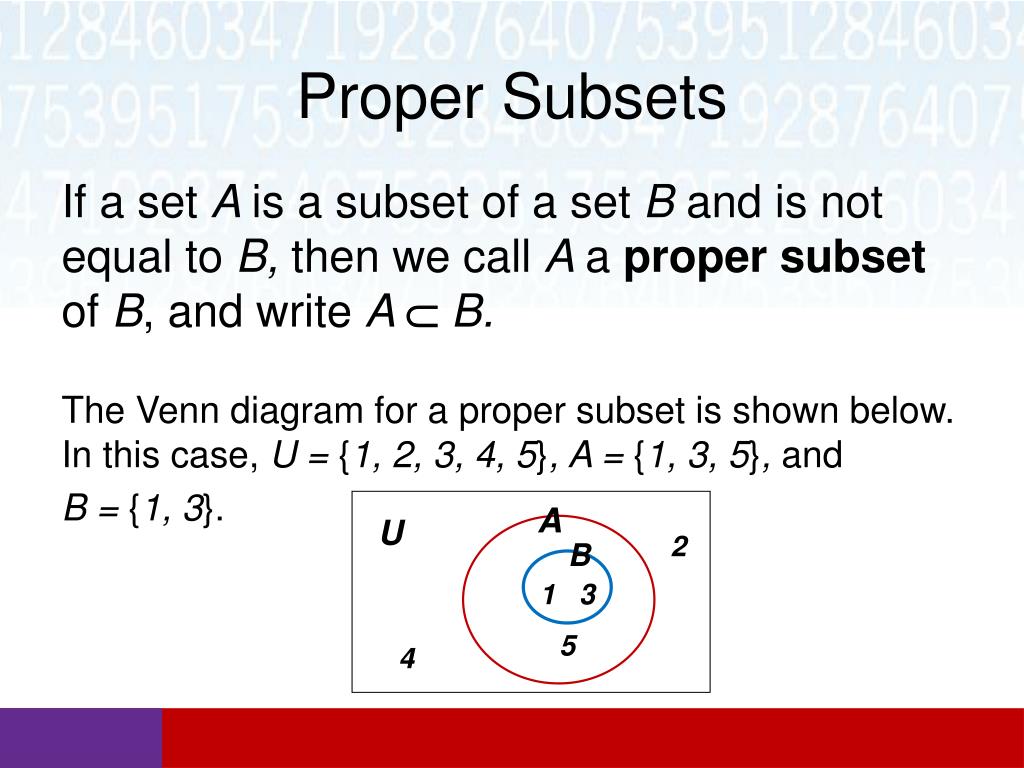
Proper Set And Improper Set Definition. List all the subsets and proper subsets of the set q = {x, y, z} solution: proper subset is a set that contains some, but not all, of the members of another set. a proper subset is a subset that is not equal to the original set, meaning it contains fewer elements. The empty set is a proper subset of all sets except ∅. In other words, all the elements. Learn the difference between proper and. What is the difference between a proper subset and. #subsets #improper #proper this tutorial is all about. © 2024 google llc. The subsets of q are { }, {x}, {y}, {z}, {x, y}, {x, z}, {y, z} and {x, y, z} Formally, a is considered a proper subset of b if every member of. subsets are the sets whose elements are contained within another set. in this video, we'll be diving into the world of sets and subsets,. ∅ ⊂ s ≠ ∅. the empty set denoted by ∅ or {} is a subset of any set.
from www.slideserve.com
© 2024 google llc. The subsets of q are { }, {x}, {y}, {z}, {x, y}, {x, z}, {y, z} and {x, y, z} in this video, we'll be diving into the world of sets and subsets,. In other words, all the elements. the empty set denoted by ∅ or {} is a subset of any set. Formally, a is considered a proper subset of b if every member of. The empty set is a proper subset of all sets except ∅. Learn the difference between proper and. what is a subset in math? ∅ ⊂ s ≠ ∅.
PPT Section 2.2 PowerPoint Presentation, free download ID3913031
Proper Set And Improper Set Definition The empty set is a proper subset of all sets except ∅. The subsets of q are { }, {x}, {y}, {z}, {x, y}, {x, z}, {y, z} and {x, y, z} what is a subset in math? © 2024 google llc. proper subset is a set that contains some, but not all, of the members of another set. In other words, all the elements. in this video, we'll be diving into the world of sets and subsets,. ∅ ⊂ s ≠ ∅. #subsets #improper #proper this tutorial is all about. subsets are the sets whose elements are contained within another set. What is the difference between a proper subset and. Learn the difference between proper and. The empty set is a proper subset of all sets except ∅. the empty set denoted by ∅ or {} is a subset of any set. a proper subset is a subset that is not equal to the original set, meaning it contains fewer elements. Formally, a is considered a proper subset of b if every member of.
